Course Websites
 |
| Paul Klee, Ad Parnassum (1932) |
| MUSC 115 | Music Theory I |
| MUSC 116 | Music Theory II |
| MUSC 215 | Music Theory III |
| MUSC 216 | Music Theory IV |
| MUSC 315 | Analysis of Tonal Music |
| MUSC 336 | Introduction to Computer Music |
| MUSC 525 | Post-Tonal Theory |
| MUSC 529 | Eighteenth-Century Counterpoint |
| MUSC 540 | Projects in Computer Music |
| MUSC 725 | Contemporary Styles II (1945-80) |
| MUSC 726A | The Art of Counterpoint |
| MUSC 726C | The Counterpoint of J.S. Bach |
| MUSC 726D | Debussy & Ravel |
| MUSC 726G | Geometrical Music Theory |
| MUSC 726T | Tuning Theory |
| MUSC 737 | Advanced Projects in Computer Music |
Courses currently in development:
| MUSC 312 MUSC 726 |
Counterpoint The Canons & Fugues of J.S. Bach |
Other courses I have taught at USC:
| SCHC 367M SCHC 367P |
Music and Science Philosophical Perspectives on Music |
| MUSC 530 | 16th-Century Counterpoint |
| MUSC 539 | Composing with Computers |
| MUSC 700 | Max Programming Mathematical Music Theory Open Music Post-Tonal Analysis Neo-Riemannian Theory |
| MUSC 715 | Performance and Analysis |
| MUSC 726 | Advanced Counterpoint Music and Mathematics Music, Acoustics, and Cognition Musical Aesthetics |
| MUSC 729 | Contrapuntal Techniques |
Resources
Lautzenheiser (1992) identifies some qualities of successful teachers:
- They are CARING.
- They show tremendous DEDICATION.
- They always HAVE TIME for their students.
- They have a good SENSE OF HUMOR.
- They can COMMUNICATE well.
- They ENJOY teaching.
- They show PERSONAL DISCIPLINE.
- They are FAIR.
- They demonstrate PERSISTENCE.
- They RESPECT their students.
— Tim Lautzenheiser, The Art of Successful Teaching
What the hand does, the mind remembers.
— Maria Montessori

The only source of knowledge is experience.
— Albert Einstein
All genuine learning is active, not passive. It involves the use of the mind, not just the memory. It is a process of discovery, in which the student is the main agent, not the teacher.
— Mortimer Adler
Active learning is "anything that involves students in doing things and thinking about the things they are doing.
— Charles Bonwell and James Eison
The role of the teacher is to create the conditions for invention rather than provide ready-made knowledge.
— Seymour Papert
...if teachers can communicate their reasons for actually loving a certain kind of music, they have rendered a useful service.
— Rose Rosengard Subotnik
...Fux truly realized that teaching means to impart learning and that in order to assume his role as interpreter of the past, the teacher himself must assume the role of disciple.
— Alfred Mann

...20 percent of the children in a certain elementary school were reported to their teachers as showing unusual potential for intellectual growth. The names of these 20 percent of the children were drawn by means of a table of random numbers, which is to say that the names were drawn out of a hat. Eight months later these unusual or 'magic' children showed significantly greater gains in IQ than did the remaining children who had not been singled out for the teachers' attention. The change in the teachers' expectations regarding the intellectual performance of these allegedly 'special' children had led to an actual change in the intellectual performance of these randomly selected children.
— Robert Rosenthal and Lenore Jacobson, Pygmalion in the Classroom

Frameworks
- Bloom's Taxonomy (Anderson, Krathwohl and Bloom 2001)
- Patricia Armstrong on Bloom's Taxonomy {Vanderbilt}
- Gardner's Theory of Multiple Intelligences (MI) (Gardner 1983)
- The components of MI {MI Oasis}
- Howard Gardner on his Theory of Multiple Intelligences (1997) {Edutopia}
- Youki Terada, Multiple Intelligences Theory: Widely Used, Yet Misunderstood (2018) {Edutopia}
- Universal Design for Learning (UDL) {CAST.org}
- Learning Guidelines {CAST.org}
Teaching & Learning Resources
- Columbia, Center for Teaching and Learning, Teaching Resources {Columbia}
- Contemplative Pedagogy
- Cornell Center for Teaching Excellence {Cornell}
- Active Learning
- Harvard's Derek Bok Center for Teaching and Learning {Harvard}
- Great Teacher Videos
- Thomas Kelly, First Nights {YouTube}
- Guides to Teaching Writing
- The Science of Learning
- Stanford Teaching Commons {Stanford}
- Blended and Hybrid Teaching Guide
- University of California, Berkeley
- Center for Teaching & Learning {Berkeley}
- GSI Teaching & Resource Center {Berkeley}
- Learning: Theory and Research
- Education: Learning to Think in a Discipline
- University of Chicago, Teaching & Learning Resources {Chicago}
- University of Illinois, Center for Innovation in Teaching and Learning {Illinois}
- University of Michigan, Center for Research on Learning and Teaching {Michigan}
- University of South Carolina, Center for Teaching Excellence (CTE) Resources {USC}
- Vanderbilt, Center for Teaching, Teaching Guides {Vanderbilt}
- Teaching Problem Solving
- Yale, Poorvu Center for Teaching and Learning {Yale}
- Active Learning {Yale} (Bonwell and Eison 1991)
Teaching Music Theory
- AP Music Theory {College Board}
- Bain, Teaching Post-Tonal Theory Bibliography {MUSC 525}
- Engaging Students: Essays in Music Pedagogy Journal: Vol. 1-6; Vol. 7-present
- Gotham, et al., Open
Music Theory, v2
- IB Music {IBO}
- Journal of Music Theory Pedagogy {App State}
- Lumsden and Swinkin, eds., The Norton Guide to Teaching Music Theory {GBd} (Lumsden and Swinkin 2018)
- Rogers, Teaching Approaches in Music Theory, 2/e {GB} (Rogers 2004)
- Society for Music Theory (SMT), Music Theory Online {MTO}
- VanHandel, ed., The Routledge Companion to Music Theory Pedagogy {GB} (VanHandel 2020)
By forming and developing a set of consistent conceptual principles and a personalized belief system for teaching theory from an awareness of the similarities/differences and strengths/weaknesses of competing systems, we simultaneously solidify our own values and open our minds and ears to additional possibilities.
— Michael Rogers, Teaching Approaches in Music Theory

Music, Science, Mathematics & Technology
- Acoustical Society of America {AcousticalSociety.org}
- American Mathematical Society {AMS.org}
- Mathematics and Music {AMS.org}
- Association for Computing Machinery (ACM): Advancing Education {acm.org}
- Association for Technology in Music Instruction {ATMImusic.com}
- Computational Thinking {CMU.edu} (Wing 2006)
- Computational thinking, 10 years later (Wing 2016) {Microsoft.com}
- HoTEL (HOlistic approach to Technology Enhanced Learning) {Hotel-project.eu}
- Richard Millwood, Learning Theory v6, a hypertextual concept map of established learning theories {Richardmillwood.net}
- IRCAM Forum {forum.IRCAM.fr}
- ISTE Standards for Educators {ITSE.org}
- Mathematics Across the Curriculum {Dartmouth}
- Mathematical Association of America {MMA.org}
- National Science Foundation {NSF.org}
- Broader Impacts {}NSF.org}; Eduction {}NSF.org}
- New Interfaces for Musical Expression {NIME.org}
- Society for Mathematics and Computation in Music (SMCM) {SMCM-net.info}
- STEM: Science, technology, engineering, and mathematics {WP}
- Wolfram for Education {Wolfram.com}
Computational thinking is a fundamental skill for everyone, not just for computer scientists.
— Jeannette M. Wing, Computational Thinking
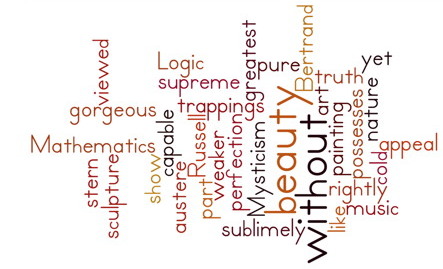
Mathematics, rightly viewed, possesses not only truth, but supreme beauty — a beauty cold and austere, like that of sculpture, without appeal to any part of our weaker nature, without the gorgeous trappings of painting or music, yet sublimely pure, and capable of a stern perfection such as only the greatest art can show.— Bertrand Russell, Mysticism and Logic
Intersections between music, mathematics, science, language, philosophy, technology, and more
- Leonard Bernstein, The Unanswered Question: Six Talks at Harvard {GB} (Bernstein 1976) James Elkins, How to Use Your Eyes. {GB} (Elkins 2000)
- Martin Gardner, Fractal Music, Hypercards, and More... {GB} (Gardner 1992) Martin Gardner Tribute in MMA Focus {MAA.org}
- James Gleick, Chaos: The Making of a New Science {GB} (Gleick 1989)
- David Goodstein, The Mechanical Universe {YouTube Playlist} (Goodstein 1985)
- Brian Greene, The Elegant Universe {GB; PBS} (Greene 2003)
- Brady Haran, Numberphile {YouTube Channel; Website}
- Douglas Hofstadter, Gödel, Escher, Bach {Wikipedia} (Hofstadter 1979)
- Susanne Langer, Philosophy in a New Key: A Study in the Symbolism of Reason, Rite, and Art {GB} (Langer 1942)
- Leonard B. Meyer, Emotion and Meaning in Music {GB} (Meyer 1956)
- Carl Sagan, Cosmos {WP}
- Grant Sanderson, 3Blue1Brown {YouTubeChannel; Website}
Advancing Communication, Teaching & Learning
- Alan Alda Center for Communicating Science {StonyBrook.edu}
- Association for Computing Machinery (ACM): Advancing Education {ACM.org}
- Carnegie Foundation for the Advancement of Teaching {CarnegieFoundation.org}
- Coursera {Coursera.org}
- Edutopia {Edutopia.org}
- EdX {Edx.org}
- Harvard, Norton Lectures {Harvard.edu}
- Kadenza: Welcome to the Future of STEAM Education {Kadenza.com}
- National Council of Teachers of Mathematics {NCTM.org}
- National Science Foundation: Education {NSF.gov}
- TED: Ideas worth spreading {TED.com}
- TED-Ed: Lessons worth sharing {TED.com}
- TED Talks on Music {TED.com}
- World Science Festival {WorldScienceFestival.com}
Art & Science in the Media
- MIT, MIT Technology Review
- Nature, Science & Music Web Focus
- New York Times: Arts | Science Times
- NPR, Science Friday
- PBS, Nova
- SETI Institute, Big Picture Science
- WNYC, Radiolab
References
Anderson, Lorin W., David R. Krathwohl, Benjamin S. Bloom. 2001. A Taxonomy for Learning, Teaching, and Assessing: A revision of Bloom's taxonomy of educational objectives. New York: Longman. {GBd}
Apostol, Tom M. and James F. Blinn. 1988-2000. Project Mathematics! Video series by PBS/NASA/CalTech. {YouTube Playlist; WP}
Bernstein, Leonard. 1976. The Unanswered Question: Six Talks at Harvard. Cambridge: Harvard University Press. {GB}
Bloom, Benjamin. 1956. Taxonomy of Educational Objectives, Handbook I: The Cognitive Domain. New York: David McKay Co Inc. {GB}
Bonwell, C., and J. Eison. 1991. "Active learning: Creating excitement in the classroom." ASHE–ERIC Higher Education Report No. 1. Washington, DC: The George Washington University, School of Education and Human Development. {Eric.ed.gov}
Burns, Ken and Lynn Novick. 1998. Frank Llyod Wright: A film by Ken Burns & Lynn Novick. PBS America. {PBS}
Calter, Paul. 2008. Squaring the Circle: Geometry in Art and Architecture. New York: Wiley. {GB}
Elkins, James. 2000. How to Use Your Eyes. New York: Routledge. {GB}
Gardner, Howard. 1983. Frames of Mind: The Theory of Multiple Intelligences. New York: Basic Books. {GB}
Gardner, Martin. 1992. Fractal Music, Hypercards, and More...: Mathematical Recreations from Scientific American Magazine. New York: SA. {GB}
Gleick, James. 1988. Chaos: The Making of a New Science. New York: Penguin. {GB}
Goodstein, David. The Mechanical Universe...And Beyond. Video series by Annenberg/CPB/CalTech. {WP}
Gotham, Mark, Kyle Gullings, Chelsey Hamm, Bryn Hughes, Brian Jarvis, Megan Lavengood, and John Peterson. 2020. Open Music Theory, Version 2. Viva Press Publishing. Available online at: https://viva.pressbooks.pub/openmusictheory/>.
Greene, Brian. 2003. The Elegant Universe: Superstrings, Hidden Dimensions, and the Quest for the Ultimate Theory. New York: Norton. {GB}
Hofstadter, Douglas. 1979. Gödel, Escher, Bach. New York: Vintage Books. {GB}
Lautzenheiser, Tim. 1992. The Art of Successful Teaching: A Blend of Content & Context. Chicago: GIA Publications. {GB}
Langer, Susanne. 1942. Philosophy in a New Key: A Study in the Symbolism of Reason, Rite, and Art. Cambridge, MA: Harvard University Press. {GB}
Lumsden, Rachel and Jeffrey Swinkin, eds. 2018. Norton Guide to Teaching Music Theory. New York: Norton. {GB; Table of Contents}
Mandlebrot, Benoit. 1983. The Fractal Geometry of Nature. New York: Holt. {GB}
Mann, Alfred. 1958. The Study of Fugue. Mineola, NY: Dover. {GB}
McKeachie, Wilbert and Marilla Svinicki. 2013. McKeachie's Teaching Tips, 14th ed. Belmont, CA: Cengage. {GB}
Papert, Seymour. 1980. Mindstorms: Children, Computers, and Powerful Ideas. New York: Basic Books. {GB}
Rogers, Michael R. 2004. Teaching Approaches in Music Theory: An Overview of Pedagogical Philosophies, 2nd ed. Carbondale, IL: Southern Illinois University Press. {GB}
Subotnik, Rose Rosengard. 1996. Deconstructive Variations: Music and Reason in Western Society. St. Paul, MN: University of Minnesota Press. {GB}
______________________. 1991. Developing Variations: Style and Ideology in Western Music. St. Paul, MN: University of Minnesota Press. {GB}
Rosenthal, Robert and Lenore Jacobson. 1968/1993. Pygmalion in the Classroom: Teacher Expectation and Pupils' Intellectual Development. New York: Crown House Publishing. {GB}
Russell, Bertrand. 1918. Mysticism and Logic and Other Essays. {Gutenberg.org}
Wilson, Edward O. 1999. Consilience: The Unity of Knowledge. New York: Vintage Books. {GB}
Wing, Jeannette M. 2010. "Computational thinking, 10 years later." Microsoft Research Blog 49/3 (March 23, 2016). {Microsoft}
_________________. 2006. "Computational Thinking." Communications of the ACM 49/3 (March 2006). {Carnegie Mellon}
Wolfram, Stephen. 2002. A New Kind of Science. Champaign, IL: Wolfram {Wolfram}
VanHandel, Leigh. 2020. The Routledge Companion to Music Theory Pedagogy. New York: Routledge. {GB}
Credit: Word clouds created with Jonathan Feinberg's Wordle {Wordle.net}
Updated: January 26, 2025